Energy Balance of the Atmospheric Boundary Layer

The energy balance of plants is closely connected to processes in the atmosphere, particularly to its radiation balance and to physical transport processes, which are typically studied in meteorology (see textbooks by Lutgens et al. (2013), Stull (1988) and Wallace and Hobbs (2006)). However, the absorption of solar energy used for metabolic processes, as well as the conversion into heat fluxes and the link to water vapour fluxes, take place close to the ground, where climate conditions may largely deviate from the conditions in the free troposphere.

Gregor Kraus (1911) was the first scientist to describe this phenomenon quantitatively on limestone sites near Wurzburg, Germany, and thus founded a new discipline of micrometeorology (see textbooks by Jones (2014), Monson and Baldocchi (2014) and Oke and Christen (2015)). Thus, micrometeorology focuses on the boundary layer of the atmosphere—the zone near the Earth’s surface where the mean wind speed is reduced in comparison with the free airstream in the upper atmosphere.

The radiation balance of the atmosphere and the energy balance at the ground surface—the habitat of plants—are strongly dependent on the composition of the atmosphere and the optical properties of its constituents. While many trace gases and air pollutants (e.g. ozone, nitrous oxide, ammonia, methane) affect plant life, water vapour, CO₂ and O₂ are the most important gases for a plant, highly relevant for its distribution, growth and fitness. Here, we will focus on the energy balance at ground level, linking radiation and sensible heat to water vapour and CO₂ in the atmosphere. Note: By definition, an energy balance is balanced (i.e. set to zero), while an energy budget can be out of balance (i.e. it can be positive or negative, or at zero).

Through the formation of clouds, water vapour influences:
- Absorption and reflection of short-wave radiation in the atmosphere, affecting the solar radiation reaching the Earth’s surface.
- Absorption and emission of long-wave radiation
- Density of the atmosphere and atmospheric transport processes, including precipitation, depending on water vapour saturation.
- Evaporation from the Earth's surface, depending on saturation deficit and on plant cover.
- Transpiration of vegetation.

Carbon dioxide influences:
- The thermal budget of the lower atmosphere by absorption and emission of long-wave radiation.
- Photosynthesis, as one of the key ingredients in this process

The radiation, as well as the energy balances at the ground, strongly depend on the optical properties of the atmosphere and its constituents, which interact via different biophysical processes (Mitchell 1989; IPCC 2013) and create rather complex interactions (Fig. 9.2a). The solar radiation (incident radiation on a horizontal surface) entering the Earth's atmosphere occurs in the short-wave range at about 6000 Kelvin (K), with maximum radiation at about a 0.6 μm wavelength (in the visible light).

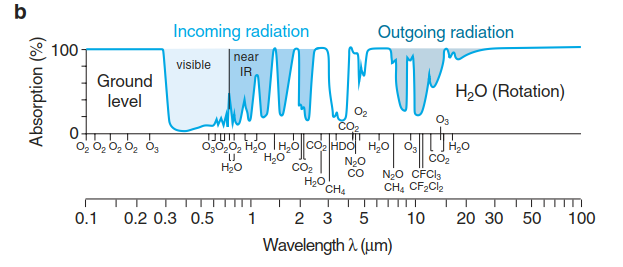
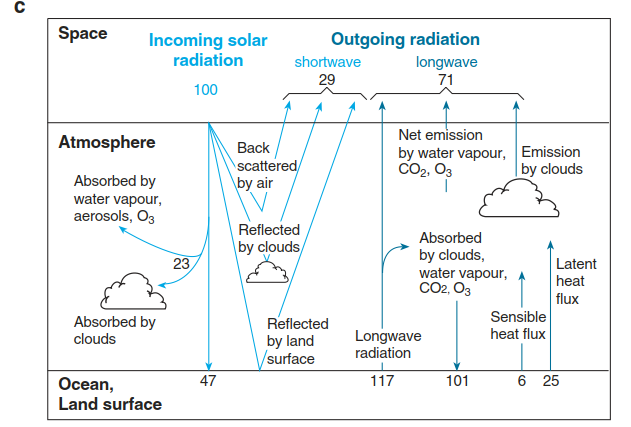
Fig. 9.2. Energy distribution and the incident radiation balance of the Earth. a Spectral distribution of short-wave incident solar radiation and long-wave thermal radiation of the Earth (Mitchell 1989). b Spectral absorption of incident radiation by gases in the atmosphere. Note that ozone absorbs short-wave radiation and ultraviolet light (UV), while CO₂ absorbs in the long-wave range. In addition, CO, N₂O and chlorofluorocarbons absorb emitted (outgoing) long-wave radiation (Mitchell 1989). c Energy balance of the Earth: transformation of incident radiation to thermal radiation at the ground surface and in the atmosphere. The percentages given are based on the average global solar incident radiation of 340 W m^-2. (Modified from Wild et al. (2013) and Hartmann et al. (2013))

The mean radiant energy flux (measured in watts per square metre or joules per square metre per second) at the upper limit of the atmosphere is about 1361 W m^-2 (solar constant, solar irradiance; measured in the stratosphere). Since the Earth is a sphere, the mean radiation flux during the day, averaged over the entire surface of the Earth, is about 340 W m^-2 (±2 W m^-2) (Wild et al. 2013). This incoming short-wave radiation (4; extraterrestrial global radiation) is partially reflected, partially balanced by outgoing long-wave radiation (thermal radiation, I₁), which by itself is a balance between thermal radiation of the atmosphere and thermal radiation from the stratosphere at a temperature of 255 K.

Long-wave radiation follows the Stefan-Boltzman law (Eq. 9.1):

where σ = 5.67 x 10^-8 (W m^-2 K^-4)—the Stefan-Boltzman constant—and T is the temperature in Kelvin. Without an atmosphere (with naturally occurring greenhouse gases), there would be no (downward, long-wave) back-radiation from the atmosphere. The average temperature on Earth would be about -18 °C (instead of about 16 °C), and thus no plant life would be possible on Earth.

However, atmospheric gases, particularly water vapour (H₂O) and CO₂, have the effect that part of the incoming solar radiation in the shortwave range is absorbed and reflected (Fig. 9.2b). Thus, the incoming short-wave radiation is limited to a narrow radiation window, with a maximum in the visible range (0.4-0.7 pm). In addition, ultraviolet (UV) radiation of the short-wave spectrum is absorbed particularly by ozone. The emitted, outgoing long-wave radiation (far-infrared) is strongly absorbed by H₂O vapour and CO₂, making the Earth hospitable for life. However, CO₂ and H₂O have an absorption minimum (emission window) between 8 and 14 pm in wavelength, in which the Earth’s surface can lose thermal energy to space. However, with increasing greenhouse gas concentrations in the atmosphere, long-wave radiation is increasingly trapped in the Earth’s atmosphere, and global temperatures are rising.

A clear distinction is made between the radiation budget and the energy balance. The radiation budget comprises the sum of all short- and longwave radiation fluxes, including their reflection, absorption or emission: incident short-wave solar radiation is absorbed and reflected by gas molecules, aerosol particles and clouds in the atmosphere (Table 9.1, Fig. 9.2c). A fraction of the incoming short-wave radiation, termed albedo (a), is reflected at the Earth’s surface, depending on the type of land or vegetation cover. Longwave radiation is emitted by the land surface and escapes into space. However, part of this emission is absorbed by clouds or trace gases, leading to back-radiation to the Earth’s surface.

Table 9.1. Energy balance of the Earth (after Hartmann et al. 2013)

Reflection, absorption and emission processes result in energy fluxes with different signs (representing the direction of the flux) and are additive in determining the energy balance of the Earth. On the other hand, the energy balance also includes—among short- and long-wave radiation fluxes—how radiation energy is dissipated into heat fluxes. These heat fluxes are the sum of thermal convection (sensible heat flux), latent heat of evapotranspiration from surfaces and of vegetation (latent heat flux), and the flux of heat into the soil (soil heat flux). While the radiation budget is normally not at equilibrium (i.e. it differs from zero), the energy budget must be zero since it is the sum of all processes (the law of conservation of energy). Thus, the energy budget is balanced (Fig. 9.2c) (Wild et al. 2013; Hartmann et al. 2013).

The net radiation budget of the Earth (R_n; n = net) may be separated into a net radiation budget at the top of the atmosphere (R_nA) and net radiation budget at the ground surface (R_nG) as expressed by the following equations:

where is the budget of radiation fluxes at the top of the atmosphere (Eq. 9.2). I_sA is the shortwave incoming (incident) solar radiation at the upper boundary of the atmosphere (extraterrestrial global radiation), p_sA is the ability of the atmosphere (clouds, gases) and the land surface to reflect incoming short-wave radiation, and I_lA is the total long-wave emission from the atmosphere (clouds, gases) and the land surface. Thus, R_nA can be calculated as 340 W m^-2 minus 100 W m^-2 minus 240 W m^-2, resulting in 0 W m^-2 at the top of the atmosphere.

The radiation budget at the ground surface may be expressed analogously. The “ground surface” is considered as the Earth’s surface, such as the soil surface or the top of a canopy. The net radiation budget at the Earth’s ground surface (R_nG) is the sum of the net short-wave radiation input to the ground and the difference between outgoing longwave emission from the land surface and back-radiation—that is, long-wave radiation re-emitted to the ground by clouds and the atmosphere (Eq. 9.3).

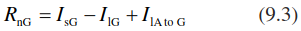
where R_nG is the budget of the radiation fluxes at the ground surface. _IsG is the net incoming shortwave radiation at the ground level, calculated as the difference between incident short-wave solar radiation (I_sA), the short-wave radiation reflected by aerosols and clouds (p_sAI_sA), and the shortwave radiation absorbed in the atmosphere. This net short-wave radiation is also often just called light. I_lG is the long-wave radiation emitted from the land surface, and I_lAtoG is the long-wave radiation re-emitted to the ground by the clouds and the atmosphere, also called back-radiation. Thus, R_nG can be calculated as 161 W m^-2 minus 398 W m^-2 plus 342 W m^-2, resulting in 105 W m^-2 at the ground surface. The net radiation budget, R_nG, is measured with a radiometer with a polyethylene dome, which is also transparent to long-wave radiation.

Moreover, the energy balance at the ground surface (i.e. at the soil or canopy surface) also includes the heat fluxes into which the net radiation at the ground is dissipated, thus balancing the energy budget (Eq. 9.4).

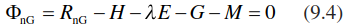
where Ф_nG is the energy balance at the ground surface (soil or canopy), and R_nG is the net radiation budget at the ground surface. The sensible heat flux, H, is proportional to the specific heat capacity of air (c_p = 1012 J kg^-1 K^-1) and the temperature difference ∆Т between the ground surface and the atmosphere, p is the density of air: (1.1884 kg m^-3 at 20 °C and 100 kPa of air pressure). H, the upward flux of sensible heat, is dependent on the coupling of the exchange from the surface to the atmosphere. This coupling is expressed by the boundary resistance for heat transfer, r_b:

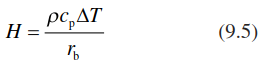
In Eq. 9.4, λE is the latent heat flux, whereby λ expresses the energy required for evaporation of water (2.454 MJ kg^-1 at 20 °C) and E is the evaporation from the soil and the transpiration of vegetation (kg m^-2 s^-1). Furthermore, Ф_nG also includes the soil heat flux—that is, the downward-oriented sensible heat flux, G (omitted in Table 9.1 because of its negligible magnitude at global level). Energy used in metabolism (M) is very small in comparison with all other energy balance components and thus is most often ignored (as in Table 9.1).

The annual incoming short-wave solar radiation (Fig. 9.3) is unevenly distributed over the Earth’s surface. Net radiation is highest over dry areas because there are fewer clouds, and it decreases towards the poles to about 40% and in the tropics to 70% of the radiation in the upper atmosphere. The global distribution of radiation is reflected in the global temperature and, in particular, low temperatures and frosts, and thus affects vegetation distribution, as well as plant performance.

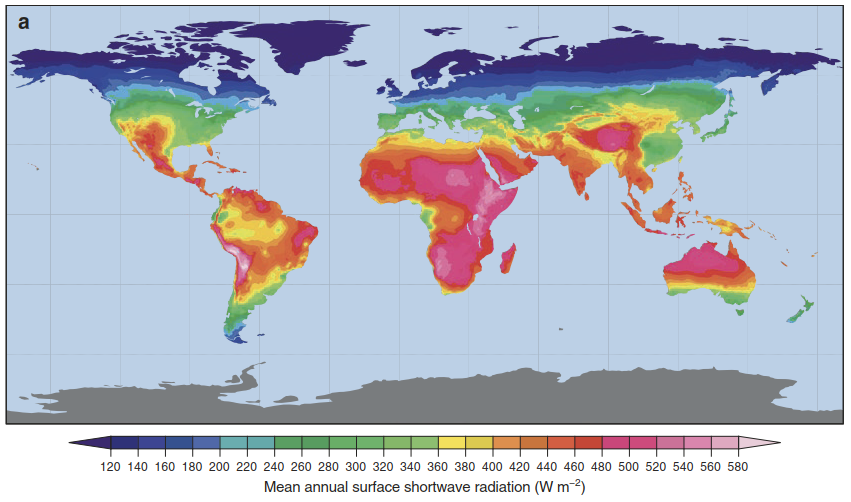
Fig. 9.3. Global distribution of solar radiation and occurrence of frosts. a Solar radiation is given as short-wave incident radiation of the sun at the Earth’s surface. b Frosts are given as absolute minimum temperatures recorded. (Images by J. Kaplan)

About 342 W m^-2 of the 398 W m^-2 long-wave emissions from the Earth’s ground surface I_lG in Table 9.1) are re-emitted to the ground by clouds and the atmosphere (I_lAtoG). This effect, also called the greenhouse effect, is due to clouds and trace gases and their effect on the outgoing long-wave radiation from the Earth. Water vapour, CO₂, methane (CH₄), nitrous oxide (N₂O) and other trace gases such as ozone and anthropogenic chlorofluorocarbons (CFCs) absorb long-wave radiation (I_lAtoG) and re-emit it towards the ground, affecting the temperature of the atmosphere of the Earth—that is, its climate (Stott et al. 2001).

These trace gases are therefore called greenhouse gases. In particular, ozone and CFCs absorb exactly at the wavelength of the maximum longwave emission by the Earth and are thus more effective than trace gases such as CO₂, CH₄ and N₂O, which decrease the outgoing radiation at the edge of the absorption spectrum (Fig. 9.2b). Further details will be discussed in Part 5. Trace gases in the Earth’s atmosphere are to a certain extent analogous to the window glass of a greenhouse. As window or greenhouse glass is more permeable to short-wave radiation than to longwave radiation, short-wave radiation passes through the window glass without resistance and is absorbed by the atmosphere and ground surfaces inside the greenhouse, leading to heating of this volume.

At the same time, long-wave radiation is emitted at the temperature of this absorbing volume, but this long-wave radiation cannot pass through the glass. It is thus “trapped” in the greenhouse. As a consequence, the temperature in the greenhouse further increases by heat conduction from the absorbing surfaces, since energy enters (as short-wave radiation) but does not exit (as long-wave radiation). However, the greenhouse effect in a greenhouse is intensified in comparison with the Earth’s greenhouse gas effect, since convective transport of the heated air masses is prevented in a closed greenhouse but is possible in the environment outside confined buildings.