Distance. The Friction of Distance. Distribution

Distance measures how far apart two places are. Combining information about distance with information about direction helps to fix relative location more accurately.

Although we measure distance on the earth's surface in units of length, we often use other measures as surrogates for length in determining distances. In ordinary conversation, we often use time as a measure of distance. A commuter may describe her residence as half an hour from her office. As any frustrated rush-hour commuter knows, time distance and linear distance often differ. An eight-mile drive during rush hour in a crowded city takes much longer than an eight-mile drive on an empty highway.

Relative distance is often measured in terms of cost, especially when long linear distances are involved. Airfares are often independent of absolute distance. Fares between large, distant cities are often lower than those between smaller, closer places. In the spring of 1991 it was substantially cheaper to fly from Miami to London. England, than to Omaha. Kansas City, or New Orleans. Lower airfares on long-distance routes between large cities reflect higher demands for service between them. Consistently low airfares reduce the relative distance between places by encouraging travel between them.

The Friction of Distance. Such measures of relative distance as length, time, and cost can be integrated by the concept of the friction of distance. The friction of distance is a measure of the difficulty associated with movement or communication between places. The greater the friction of distance, the more difficult it is to overcome distance.

In recent decades, improvements in transportation and communications technology have considerably reduced the friction of distance in the modern world. This decline in the friction of distance is called time-space convergence (Figure 1-4).

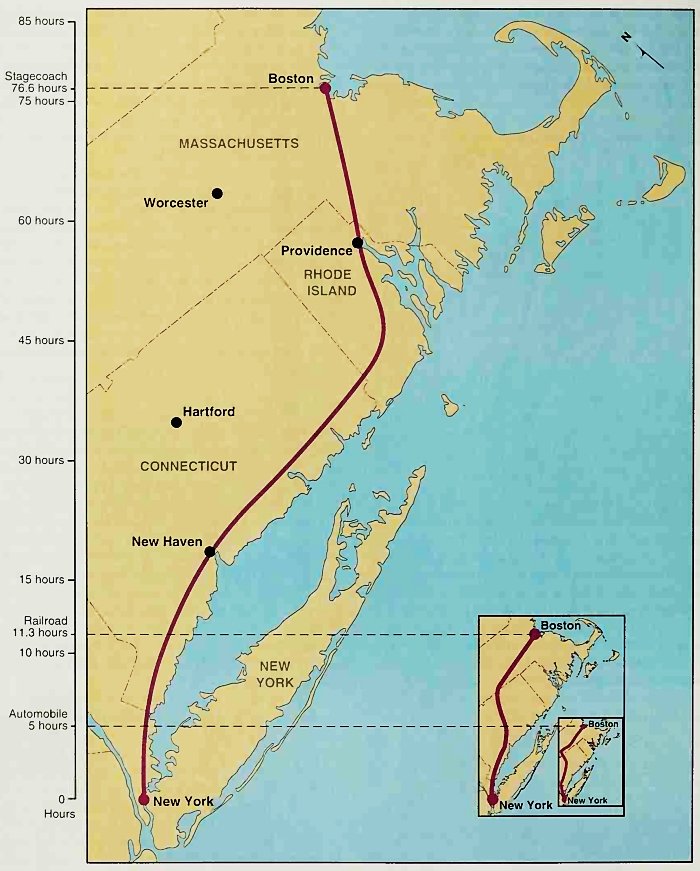
Figure 1-4. Time-Space Convergence. The relative distance between most pairs of places has steadily decreased in accordance with the development of modern technology, as illustrated by this example showing changes in travel time between New York and Boston. Today, it is possible to communicate with people throughout the world instantaneously

In the early nineteenth century, a trip between New York and Los Angeles required several months. The traveler could choose between a hazardous overland journey on foot, horseback, or stagecoach and a long ocean trip. Ocean travelers sailed to Panama, crossed dense jungles and steep mountains by land near the site of the present-day Panama Canal, and finally embarked on ships sailing along the Pacific coast to Los Angeles.

The completion of the transcontinental railroad in 1869 reduced the journey across North America from several months to a few days. Jet aircraft now make the trip in four or five hours. Communications have converged in space and time even more quickly. In the early nineteenth century, messages could travel no faster than human carriers. The invention of the telegraph, telephone, radio, and television revolutionized long-distance communication. Today, messages can be sent from New York to Los Angeles immediately via facsimile machine, electronic mail, or telephone.

Distribution. How things are arranged on the earth's surface is another fundamental concern of human geography. Why do certain events take place in specific locations and not else-where? Why are events concentrated in some places and absent in others? At what locations were casualties and major damage episodes concentrated following the San Francisco earthquake?

In answering questions such as these, geographers are concerned with distributions. The distribution of any occurrence is its arrangement on the earth's surface or a portion of the earth's surface. Geographers examine maps of distributions in order to understand why phenomena are located where they are.

The geometric characteristics of distributions help the geographer to interpret and explain them. Two of the most important geometric characteristics of distributions are pattern and density. The pattern of a distribution—its geometric arrangement in space—can be random, regular, linear, or clustered (Figure 1-5).

Figure 1-5. Random. Regular, and Clustered Patterns. Distributions can be random (a), regular (b), or clustered (c). Whereas regular distributions are evenly spaced, clustered distributions are characterized by objects or events in a single location or a small number of places. In random patterns, the location of one point has no relationship to that of another

Density is the frequency of a phenomenon per unit of land area. It is measured by dividing the frequency of occurrence by the land area in question. For example, population density is the number of people per square mile or square kilometer.

Density values are valuable in many research contexts because they are easily mapped and compared. Analysis of densities is especially useful in research in which large and small places are compared. The population of the Netherlands is much smaller than that of France, yet the population density of the Netherlands is much greater because its land area is so much smaller.