To measure board and timber by arithmetic, being equal or unequal, and to find how many inches in length makes a foot of any breadth

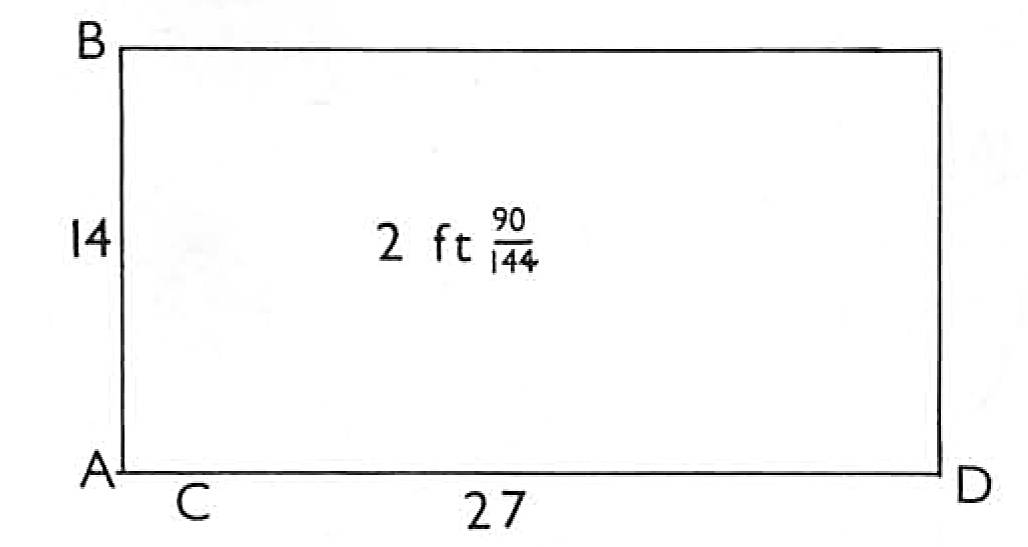
To measure any board, stone, glass, or any measure by arithmetic. I shall be very brief in every part of this work, showing one example to every way of measure, by which all others are performed, and therefore I shall begin with a board or glass, as supposing a board 14 inches broad from A to Band 27 inches long from C to D. I desire to know how many feet square of board is in this figure. Work thus.

Multiply 14 inches, which is the breadth, by 27 inches, which is the length, and it produceth

378. Having so done, divide this 378 by 144 and it will give the quotient 2 90/144 which is 2 feet and 90 square inches, or 90 parts of 144, which is very near two thirds of a foot, so that there is 2 feet and near two thirds in this flat board, whose breadth is 14 inches and length 27 inches. Now the reason of your multiplying the breadth by the length is to produce the quantity of inches, which is 378.

If you strike 14lines in the breadth and 27 lines in the length will produce 378 single parts, and why you divide it by 144 is that 12 lines being struck each way will be 144, and 12 inches being a foot and divided 12 times will produce the same for a divisor. So that, these reasons being observed, you are master of all flat measure, the multiplication, bringing all into inches, the division into feet square, which was required to be known. In like manner is all flat measure wrought, whatsoever.

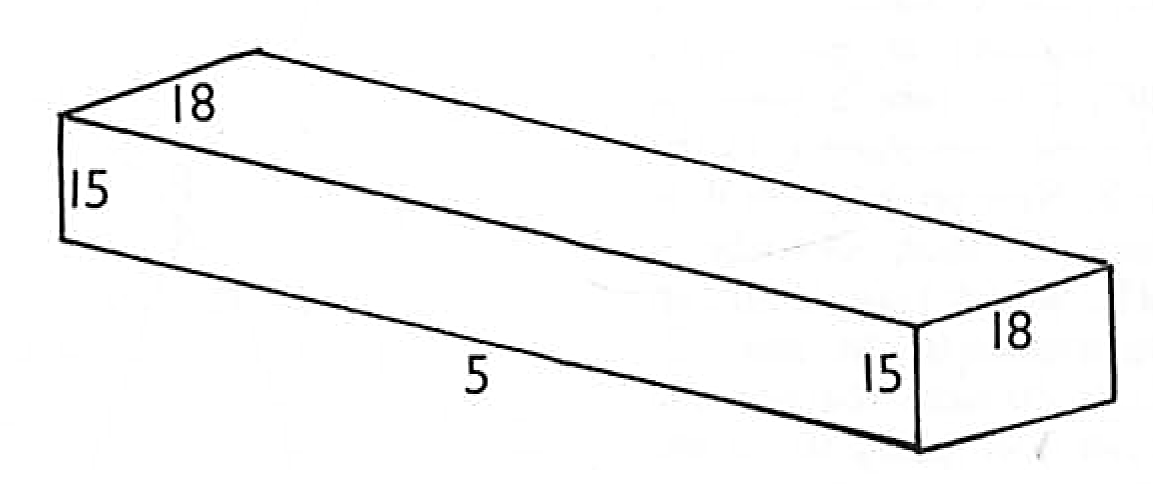
To measure any square timber by arithmetic, as suppose a piece of timber which is 15 inches broad and 18 inches thick, and 5 feet long. I desire to know the square feet of timber contained in

this piece. Work thus. Multiply the side 15 by the thickness 18, and that produceth 270. Having so done, turn the 5 feet which is the length of the piece intoinches, by multiplying it by 12. It will

give 60 inches long. Now have you the length and breadth in inches. Then multiply 270, your square of the piece, by the length 60, and it yields 16200. Having so done, divide this 16200 by 1728 and the quotient will be 9 648/1728 feet, the true content of the piece above mentioned.

The reason why it is thus multiplied is to bring the whole piece into square inches, and why the divisor is 1728 is by reason so many square inches is in a foot square, as in the last chapter appears. For 12 inches flat has 144 upon one side, therefore it must be 12 times 144, if it be

a foot thick as well as breadth, which was required to be made manifest. In this manner is all timber measured which hath breadth and thickness, but you will find some pieces of many sides

equal or unequal, and round, all of which you shall find in their due places as we proceed. And by the way, remember that every 12 inch square of board hath 144 inches, but every foot square of timber hath 1728 inches, being 12 times so much by reason of the thickness.

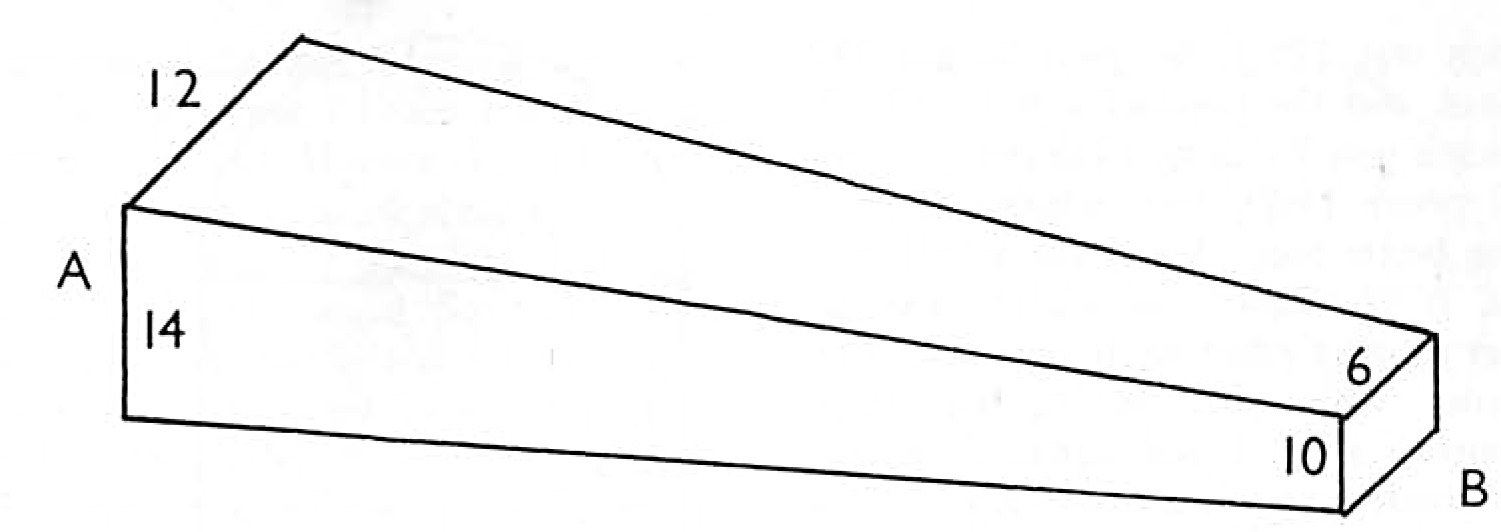
To measure a piece whose sides and ends are unequal, as suppose a piece whose end A is 14 inches broad and 12 inches thick, and his end B 10 inches broad and 6 inches thick, being 24 inches long. I desire to know the content in square feet. This piece being so irregular, both sides and ends, you must find a mean diameter 1 for its square, which do by taking the half of 10 and 14, which is 12, and the half of 12 and 6 which is 9, then multiply 12 by 9 and it produceth

108. Having so done, take the difference which is between 14 and 10, which is 4, and the half of the difference of the other side, which is 12, and 6, whose half is 3, and multiply them one into the other, saying 3 times 4 makes 12, then divide 12 by 6 and it produceth 2 in the quotient, and add this 2 to the 108. It makes 110.

Out of this 110 extract the square root, and the quotient will be 10 1/2 inches, very near, for your mean diameter of this piece. Now multiply 10 1/2 by 10 1/2 . The product will be 110. This 110 multiply by 24 inches, being the length of the piece, and it produceth 2640. Then divide this 2640 by 1728 and the quotient will be 1 912/1728 feet.

Why I take these means, observe that when you had taken the difference between the ends there remains a pyramid on the side, whose content was 2 inches, which I added to the former, and why divided by 6, is by reason every pyramid is the 6th part of a cube whose bases are equal, as you will find in its due place. And therefore I shall say no more, having showed you the hardest way of timber measure, which being observed you may resolve any question whatsoever.

To find how many inches in length will make a foot of square timber. By this method is graduated the carpenter's ruler, which discovers how many inches in length makes a foot of any square measure, as for example I would know how many inches in length will make a foot of a piece being 18 inches square. You must multiply 18 by 18 and it produceth 324. This 324 must be the divisor, and see how many times it can be had in 1728, which are the inches contained in a cube foot of timber, and your quotient will prove 5 108/324 which is 5 inches and a third of an inch in length, which makes a foot of square timber, and thus is the whole rule made. Take

another example.

I would know how many inches in length would make a foot at 12 inches square. You must work

as above said, multiplying 12 by 12, makes 144 for your divisor. Now see how many times 144 can be had in 1728, and your quotient will be 12, which is the true length of a foot of timber whose sides are 12 inches each way.

By these I suppose you well understand the making or graduating the common measure or carpenter's rule, both for board and timber, always remembering that the square inches of every foot of board, being 12 times divided and is 144, and then by consequence every piece of timber which is 12 inches thick must be 12 times 144, which is 1728, as is demonstrated in the first chapter in this folio. Now having showed you, this method, and which well observed needs no more examples.