Average Specific Gravity. Determination of Specific Gravity

Most people from everyday experience have acquired a sense of relative weight even in regard

to minerals. For example, ulexite (G 1.96) seems light, whereas barite (G 4.5) seems heavy for nonmetailic minerals. This means that one has developed an idea of an average specific gravity or a feeling of what a nonmetailic mineral of a given size should weigh. This average specific gravity can be considered to be between 2.65 and 2.75.

The reason for this is that the specific gravities of quartz (G 2.65), feldspar (G 2.60-2.75), and calcite (G 2.72), the most common and abundant nonmetailic minerals, fall mostly within this range. The same sense may be developed in regard to metallic minerals: graphite (G 2.23) seems light, whereas silver (G 10.5) seems heavy. The average specific gravity for metallic minerals is about 5.0, that of pyrite. Thus, with a little practice, one can, by merely lifting specimens, distinguish minerals that have comparatively small differences in specific gravity.

Determination of Specific Gravity. In order to determine specific gravity accurately, the mineral must be homogeneous and pure, requirements frequently difficult to fulfill. It must also be compact with no cracks or cavities within which bubbles or films of air could be imprisoned. For normal mineralogic work, the specimen should have a volume of about one cubic centimeter. If these conditions cannot be met, a specific gravity determination by any rapid and simple method means little.

The necessary steps in making an ordinary specific gravity determination are, briefly, as follows: the mineral is first weighed in air. Let this weight be represented by W_a. It is then immersed in water and weighed again. Under these conditions it weighs less, because in water it is buoyed up by a force equivalent to the weight of the water displaced. Let the weight in water be represented by W_w. Then W_a - W_w equals the apparent loss of weight in water, or the weight of an equal volume of water. The expression W_a/W_a - W_w) will therefore yield a number which is the specific gravity.

Jolly Balance. Because specific gravity is merely a ratio, it is not necessary to determine the absolute weight of the specimen but merely values proportional to the weights in air and in water. This can be done by means of a Jolly balance (Fig. 2.21), with which the data for making the calculations are obtained by the stretching of a spiral spring.

Fig. 2.21. Jolly balance

In using the balance, a fragment is first placed on the upper scale pan and the elongation of the spring noted. This is proportional to the weight in air (W_a). The fragment is then transferred to the lower pan and immersed in water. The elongation of the spring is now proportional to the weight of the fragment in water (W_w)_.

When the mineral is weighed immersed in water, it is buoyed up and weighs less than it does in air; this weight loss is equal to the weight of water it displaces. Hence, if one finds first the weight of a mineral fragment on a pan of the balance in air, and subsequently its weight while immersed in water (it being suspended on a pan by a thin wire thread), one subtracts the two weights. The difference is the weight of the equal volume of water. For example, the weight of a small quartz fragment is 4.265 grams in air; in water it is 1.609 grams. The loss of weight, or weight of an equal volume of water exactly to it, is therefore 2.656 grams; hence the specific gravity is

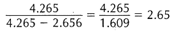
which is indeed that of quartz. In other words, the specific gravity of a mineral (G) can be expressed as follows

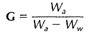
in which W_a is the weight in air and W_w is the weight in water.

Heavy Liquids. Several liquids with relatively high densities are sometimes used in the determination of the specific gravity of minerals. The two liquids most easily used are bromoform (G 2.89) and methylene iodide (G 3.33). These liquids are miscible with acetone (G 0.79), and thus, by mixing, a solution of any intermediate specific gravity may be obtained. A mineral grain is introduced into the heavy liquid and the liquid is diluted with acetone until the mineral neither rises nor sinks. The specific gravity of the liquid and the mineral are then the same, and that of the liquid may be quickly determined by means of a Westphal balance.

Heavy liquids are frequently used in the separation of grains from mixtures composed of several constituents. For example, a separation of the constituent mineral grains of a sand composed of quartz (G 2.65), tourmaline (G 3.20), and garnet (G 4.25) could be quickly made. In bromoform the quartz would float and the tourmaline and garnet would sink; they can be separated from quartz using a separatory tunnel. After removing and washing these "heavy minerals" in acetone, they could be separated from each other in methylene iodide; the tourmaline would float and the garnet would sink.