Chemistry in Aqueous Systems. Water Autoionization

Brönsted-Lowry Acid-Base Reactions . Brönsted-Lowry (BL) acids and bases are now defined using Figure 2, which illustrates the transfer of a hydrogen ion (H+) between hydrogen fluoride (HF) and ammonia (NH₃) molecules. In this reaction, HF serves in the role of the H+ ion donor, which BL theory identifies as the acid. The NH₃ serves as the H+ ion acceptor, the BL base.

Reactions involving H+ transfer are typically reversible with extremely fast kinetics in both directions, but for reasons that are beyond the scope of this article. Such systems rapidly achieve states of dynamic equilibrium.

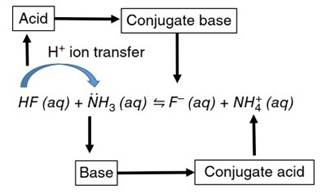
Figure 2. Brönsted-Lowry acid-base (H+ ion transfer) reaction between HF and NH₃, which yields the products base F^- and NH₄+ (i.e. the conjugate base and acid to HF and NH₃, respectively)

The HF and NH₃ molecules undergo simple and easily understood changes due to their loss and gain, respectively, of the transferred H+ ion. The resulting forms into which they are converted (F^- and NH₄+) are referred to as their “conjugate" (or, linked) forms. The acid, having lost the H+ ion, exists on the product side of the reaction in a conjugate form that is basic (i.e. capable of reaccepting an H+ ion). Thus, the F^- ion is the conjugate base of HF, and the ammonium (NH₄+) ion is the conjugate acid of NH₃.

Further points are now explicitly made about the requisite structural features that ions or molecules must possess to serve as BL acids or bases. Clearly, to serve as a BL acid, a chemical species must possess an “ionizable" H atom that is constrained by a polar covalent bond, which is predisposed toward heterolytic cleavage. Also, H+ ion transfer must convert the acid into a relatively stable conjugate base.

Conjugate bases are stable when their structures contain one or more electronegative atoms and/or they have electronic delocalization, i.e. conjugation. Conversely, a BL base must be able to expand its valence shell and form a new covalent bond as it hosts the H+ ion transferred to it. To do so, the base needs to have at least one pair of nonbonding electrons available for sharing.

The products of BL reactions occurring in aqueous solution are further stabilized via the formation of solvation spheres in which the BL product species is surrounded by water molecules. These solvation spheres exhibit H-bonding and/or dipole-dipole and/or ion-dipole intermolecular forces.

Water Autoionization. The close hydrogen bonding interaction between a pair of H₂O molecules (shown in Figure 3b) is precursory to the transfer of an H+ ion (i.e. Brönsted-Lowry reaction) between them. The H⁺ ion transfer, depicted in Figure 3a, yields the products H₃O⁺ (hydronium) and OH^- (hydroxide) ions (structures shown in Figure 3c). These ionic products are the conjugate acid and base to the water molecules that exhibited basic and acidic behavior, respectively.

Figure 3. (a) The autoionization reaction of water, which produces the product hydronium (H₃O⁺) and hydroxide (OH^-) ions. Structures of (b) the reactant pair of H-bonded H₂O molecules, and (c) the product ions. Waters of solvation not shown for clarity

The autoionization reaction possible ensues from the fact that water molecules have a dual nature. Water molecules are able to function as BL acids because they possess ionizable H atoms attached to electronegative O atoms, and the electronegative O atoms stabilize the electron density that accumulates on them when their O-H bonds are heterolytically cleaved.

Also, H₂O molecules are able to act as BL bases because they have two lone electron pairs centered on their O atoms, which allows them to form polar covalent bonds with the H⁺ ions they accept. Hence, during an autoionization process, one water molecule behaves as a BL acid and the other behaves as a BL base, (Figure 3a). Chemical species that are able to donate or accept H⁺ ions are said to be “amphoteric.’’

The form of the equilibrium constant for the autoionization reaction of water is presented in, Eq. (1), along with its value at 25 °C. This expression is an approximation assuming that the thermodynamic activities of H₃O+ and OH^- are equal to their concentrations. Activities account for nonideality that results when the ionic strength of the solution is high. It is further assumed that the activity of water is taken to be 1. These are very good approximations for pure water.

K_w (25 °C) = [H₃O+][OH^-] = 1.0 x 10^-14 (1)

Based on the small size of the equilibrium constant (K_w), this equilibrium lies mainly on the reactant side, greatly favoring intact H₂O molecules. In fact, simple calculations show that only about one water molecule in 500 million is dissociated at any given time. It is also readily seen that the concentrations of the product H₃ O+ and OH^- ions in pure water are equal, consequent to the 1 : 1 stoichiometry in Figure 3a. The concentrations in which H₃O+ and OH^- ions exist in pure water (with T = 25 C) are straightforwardly determined via a calculation based on Eq. (1). When the variable x is used to represent the molarities of the product ions, Eq. (1) changes to the form (1a).

[H₃O+][OH^-] = x² = 1.0 x 10^-14 (1a)

Hence, it is found (simply by taking the square root of each side of Eq. (1a) that x = [H₃O+] = [OH^-] = 1.0 x 10^-7 M.

It is interesting to consider the reverse direction of the autoionization reaction, which is the recombination of H₃O⁺ and OH^- ions to form water molecules (Eq. (2)). This reaction conversely has a very large equilibrium constant, which is simply the reciprocal of that for the autoionization equilibrium.

As is discussed in a later section, this reaction (with its large, product-favoring equilibrium constant) provides the fundamental driving force for most neutralization reactions through which acids and bases react with and annihilate each other.